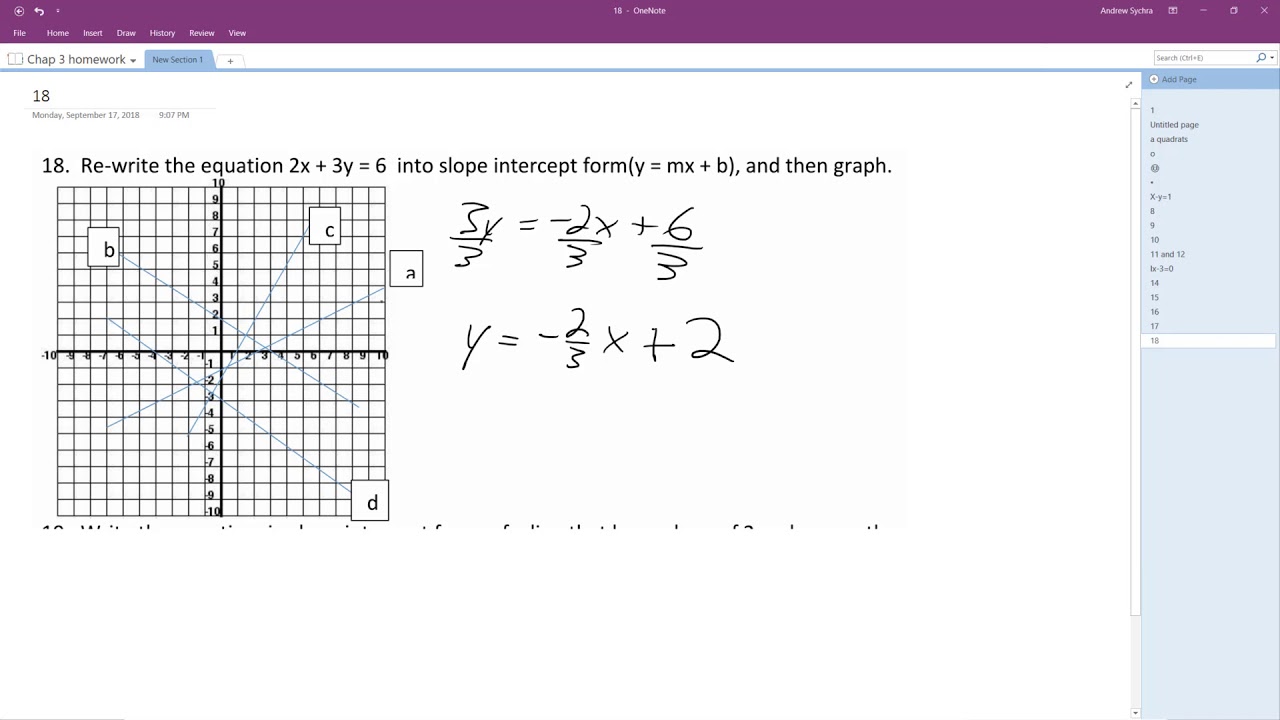
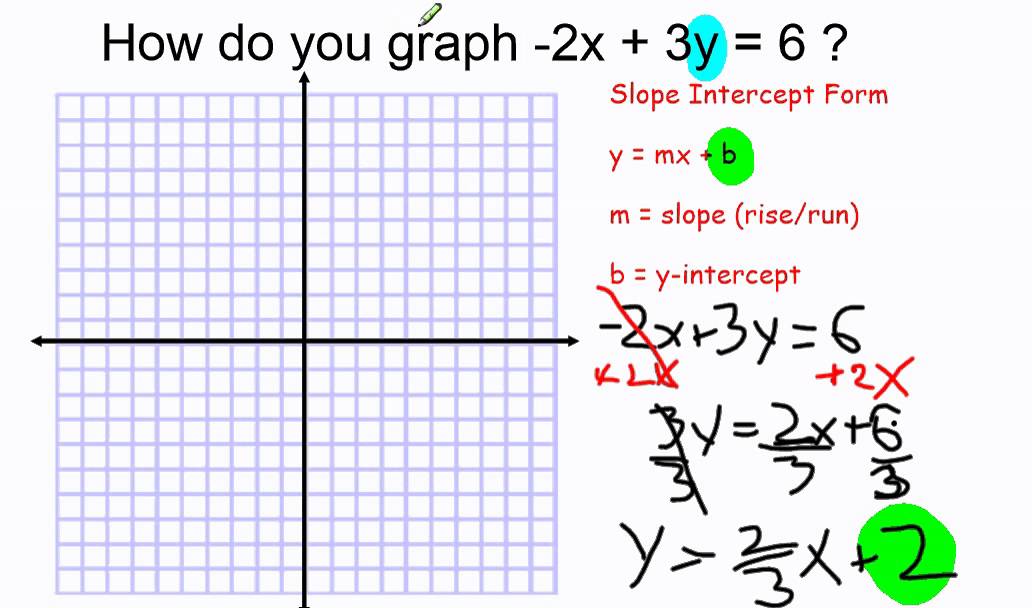
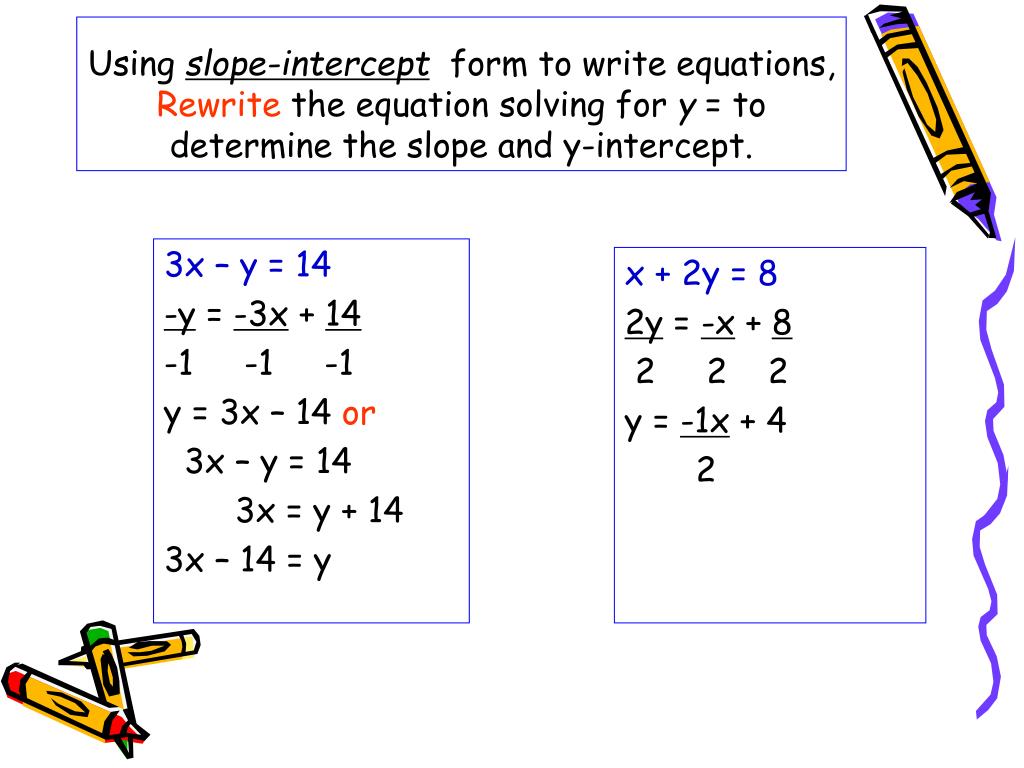
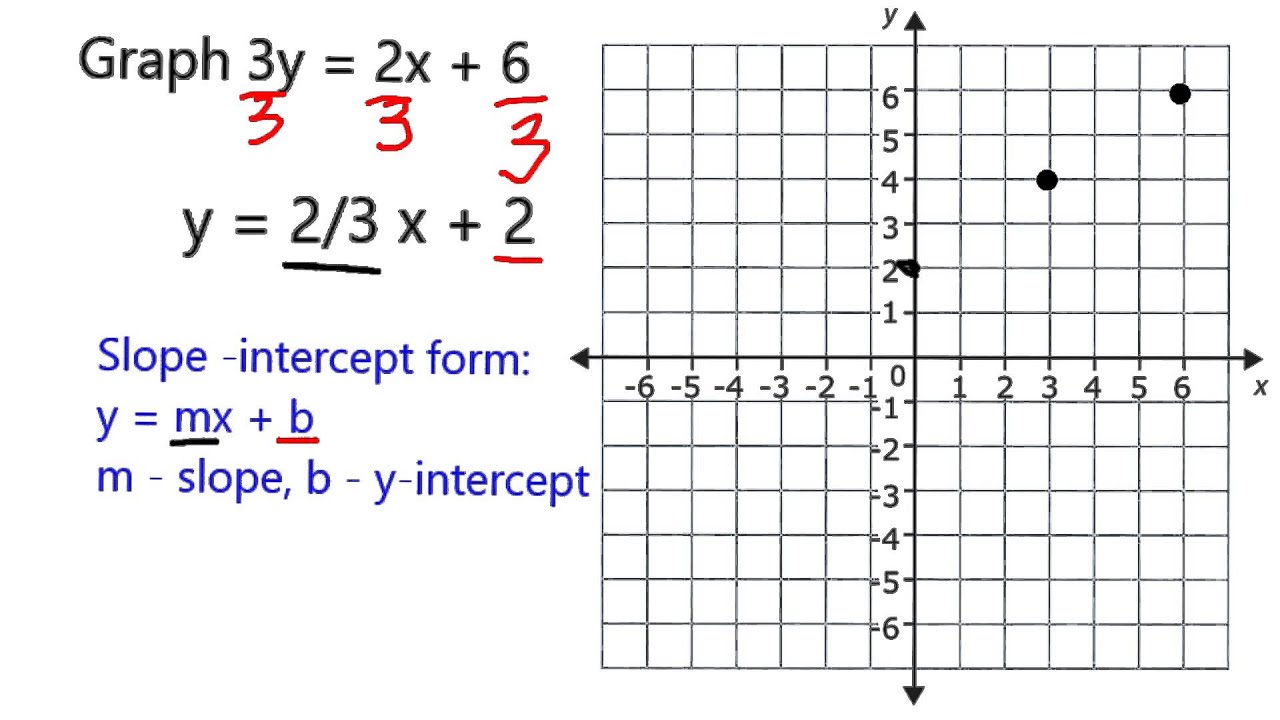
2X 3Y 9 In Slope Intercept Form
2X 3Y 9 In Slope Intercept Form - Subtract from both sides of the equation. (0,3) ( 0, 3) any line can be graphed using two points. 2x +3y = 9 3y = −2x + 9 y = − 2 3 x +9 so the slope or gradient. So we get our equation into this form: Y = 2 3x− 3. Select two x x values, and plug them into the equation to find the corresponding y y values. A straight line graph is of the form: The slope is further given by the. Subtract 2x from both the sides of the equation 2x + 3y = 9. Subtract from both sides of the equation.
M = 2 3 m = 2 3 Y = mx + c m is the gradient c is the intercept which is the point at x = 0 where the line cuts the y axis. (0,3) ( 0, 3) any line can be graphed using two points. Web to find the slope of a line, we find the rate at which the value of 'y' is increasing as we increase the value of 'x' by one unit. Rearrange the equation by subtracting what is to the. Select two x x values, and plug them into the equation to find the corresponding y y values. Y = 2 3x− 3. In slope intercept form 3y = 2x + 9 y = ( 2x + 9)/3 y = 2/3 x + 3 (a) parallel to the given line, and (b) perpendicular to it. A straight line graph is of the form:
In slope intercept form 3y = 2x + 9 y = ( 2x + 9)/3 y = 2/3 x + 3 M = 2 3 m = 2 3 So we get our equation into this form: Y = 2 3x− 3. 2x +3y = 9 3y = −2x + 9 y = − 2 3 x +9 so the slope or gradient. Rearrange the equation by subtracting what is to the. A straight line graph is of the form: (0,3) ( 0, 3) any line can be graphed using two points. Divide each term in by and. (a) parallel to the given line, and (b) perpendicular to it.
Write In Slope Intercept Form 5x 3y 9 slideshare
(a) parallel to the given line, and (b) perpendicular to it. Subtract 2x from both the sides of the equation 2x + 3y = 9. Subtract from both sides of the equation. Divide each term in by and. Rearrange the equation by subtracting what is to the.
x+3y= 9 In slope intercept form Brainly.in
Subtract from both sides of the equation. Rearrange the equation by subtracting what is to the. They've given me a reference line, namely, 2x − 3y = 9; M = 2 3 m = 2 3 Divide each term in by and.
Graph 2x + 3y = 6 YouTube
(a) parallel to the given line, and (b) perpendicular to it. Web to find the slope of a line, we find the rate at which the value of 'y' is increasing as we increase the value of 'x' by one unit. Divide each term in by and. A straight line graph is of the form: They've given me a reference.
📈write the slope intercept form of the equation for the line.Y=2x1Y=1
The slope is further given by the. M = 2 3 m = 2 3 Subtract 2x from both the sides of the equation 2x + 3y = 9. (a) parallel to the given line, and (b) perpendicular to it. Subtract from both sides of the equation.
Write In Slope Intercept Form 5x 3y 9 slideshare
Subtract from both sides of the equation. Divide each term in by and. Subtract 2x from both the sides of the equation 2x + 3y = 9. In slope intercept form 3y = 2x + 9 y = ( 2x + 9)/3 y = 2/3 x + 3 Y = 2 3x− 3.
PPT Slopeintercept Form PowerPoint Presentation, free download ID
2x +3y = 9 3y = −2x + 9 y = − 2 3 x +9 so the slope or gradient. A straight line graph is of the form: (a) parallel to the given line, and (b) perpendicular to it. The slope is further given by the. Divide each term in by and.
Use The Slope Intercept Form To Graph Equation 2x 3y 6 Tessshebaylo
Web to find the slope of a line, we find the rate at which the value of 'y' is increasing as we increase the value of 'x' by one unit. Select two x x values, and plug them into the equation to find the corresponding y y values. The slope is further given by the. M = 2 3 m.
SOLUTION How would you graph 2x + 3y = 9, and solve it into y=mx=b?
Divide each term in by and. So we get our equation into this form: Rearrange the equation by subtracting what is to the. In slope intercept form 3y = 2x + 9 y = ( 2x + 9)/3 y = 2/3 x + 3 They've given me a reference line, namely, 2x − 3y = 9;
change 2x+3y=1470 to to slope intercept form. identify the slope and
(a) parallel to the given line, and (b) perpendicular to it. Divide each term in by and. In slope intercept form 3y = 2x + 9 y = ( 2x + 9)/3 y = 2/3 x + 3 The slope is further given by the. M = 2 3 m = 2 3
Write In Slope Intercept Form 5x 3y 9 slideshare
Select two x x values, and plug them into the equation to find the corresponding y y values. Divide each term in by and. Subtract 2x from both the sides of the equation 2x + 3y = 9. 2x +3y = 9 3y = −2x + 9 y = − 2 3 x +9 so the slope or gradient. Y.
Divide Each Term In By And.
They've given me a reference line, namely, 2x − 3y = 9; Subtract from both sides of the equation. The slope is further given by the. (0,3) ( 0, 3) any line can be graphed using two points.
2X +3Y = 9 3Y = −2X + 9 Y = − 2 3 X +9 So The Slope Or Gradient.
M = 2 3 m = 2 3 In slope intercept form 3y = 2x + 9 y = ( 2x + 9)/3 y = 2/3 x + 3 Subtract 2x from both the sides of the equation 2x + 3y = 9. A straight line graph is of the form:
So We Get Our Equation Into This Form:
Y = mx + c m is the gradient c is the intercept which is the point at x = 0 where the line cuts the y axis. Y = 2 3x− 3. Divide each term in by and. Select two x x values, and plug them into the equation to find the corresponding y y values.
(A) Parallel To The Given Line, And (B) Perpendicular To It.
Subtract from both sides of the equation. Web to find the slope of a line, we find the rate at which the value of 'y' is increasing as we increase the value of 'x' by one unit. Rearrange the equation by subtracting what is to the.