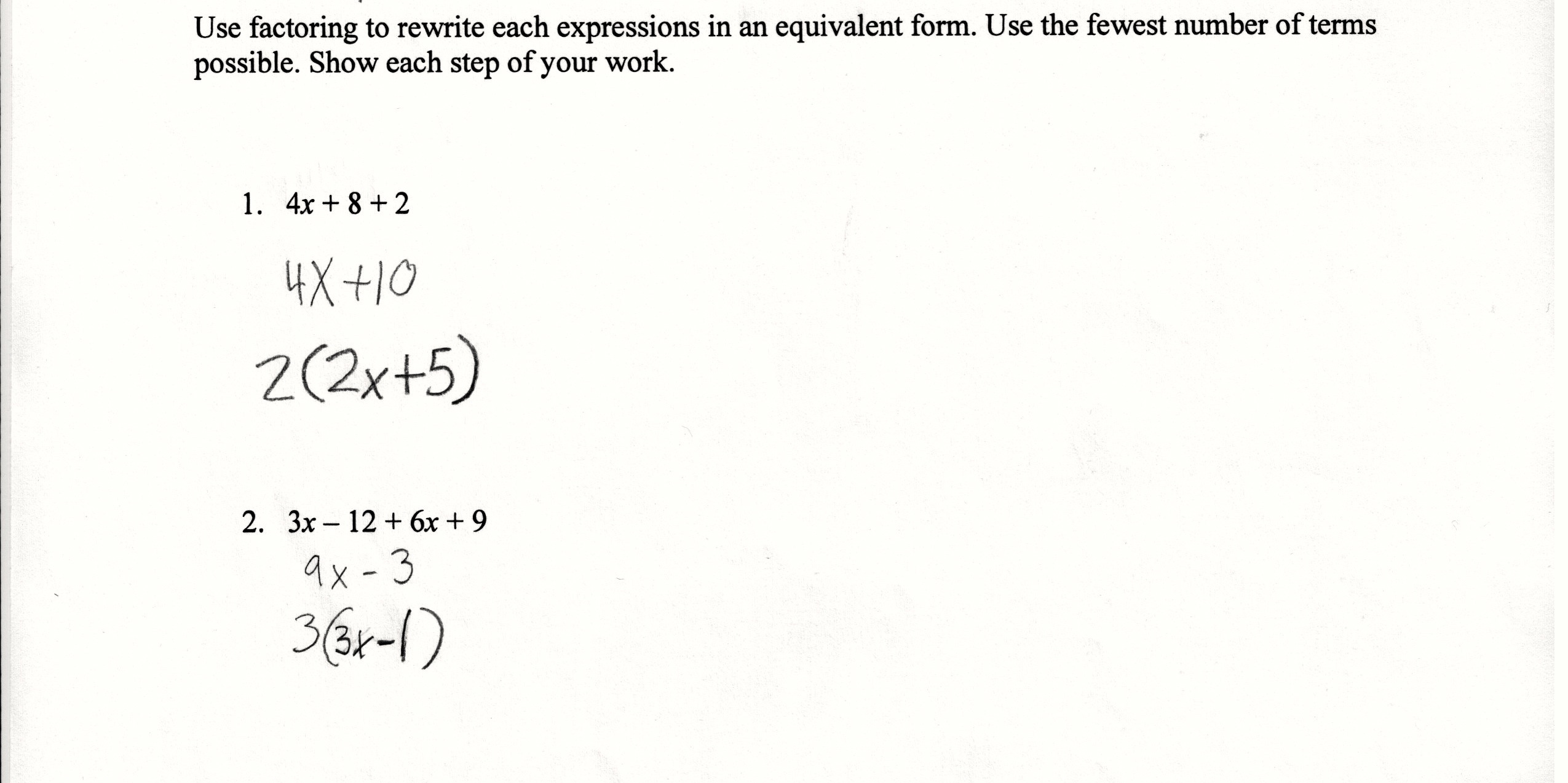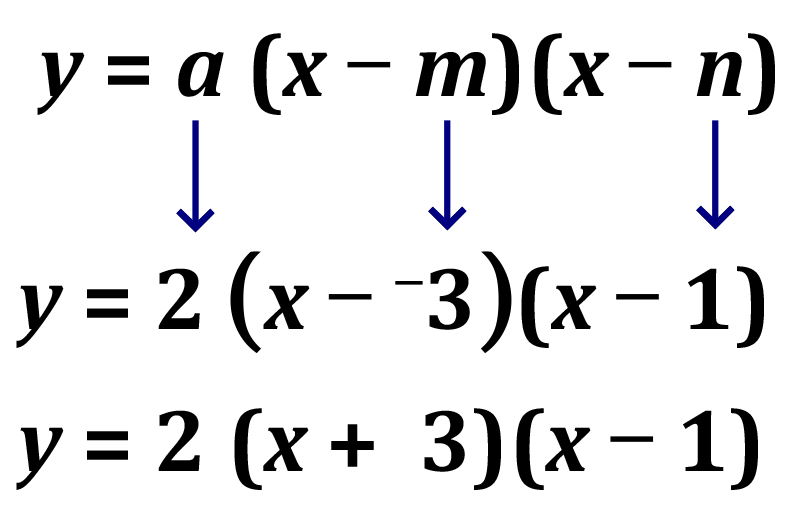What Is The Factored Form Of N 2 N
What Is The Factored Form Of N 2 N - N ⋅ (n −1)(n − 2) (n − 3)! = n(n −1)(n − 2).1. If you divide the whole thing by n. Web factor (n − (− 2 − 1)) (n − ( 2 − 1)) steps using the quadratic formula steps using direct factoring method view solution steps evaluate n2 + 2n − 1 quiz polynomial n2 +2n−1. N2 − n − 72. Depending upon the case, a suitable method is applied to find the factors. While there isn't a simplification of (2n)! Web answer (1 of 4): And calculated by the product of integer numbers from 1 to n. We can write it as:
Web answer (1 of 4): N!, there are other ways of expressing it. How do you factor a trinomial? Web factorial (n!) the factorial of n is denoted by n! Depending upon the case, a suitable method is applied to find the factors. Find a pair of integers whose product is c and whose sum is b. If you divide the whole thing by n. = (n + 2)(n + 1)n! We can write it as: Assume n =2a(2k + 1) n = 2 a ( 2 k + 1) for some integer a a and k k.
We can write it as: Trying to factor by splitting the middle term. In this case, whose product is. Web to factor a binomial, write it as the sum or difference of two squares or as the difference of two cubes. And calculated by the product of integer numbers from 1 to n. Web factorial (n!) the factorial of n is denoted by n! Web answer (1 of 4): = (n +2)(n + 1)(n)(n −1).1. N2 − n − 72. You will see that n^2/n =n.
intro to factored form YouTube
N2 − n − 72. Web factor (n − (− 2 − 1)) (n − ( 2 − 1)) steps using the quadratic formula steps using direct factoring method view solution steps evaluate n2 + 2n − 1 quiz polynomial n2 +2n−1. We can write it as: (n − 2) (n −. Find a pair of integers whose product is.
3.5 Graphing with factored form YouTube
If you divide the whole thing by n. = (n +2)(n + 1)(n)(n −1).1. Rewrite 25 25 as 52 5 2. Web factorial (n!) the factorial of n is denoted by n! We can write it as:
Factored Forms
While there isn't a simplification of (2n)! The first term is, n2 its coefficient is 1. Rewrite 25 25 as 52 5 2. We can write it as: Therefore n (n+1) arrow right.
Factored Form
= (n + 2)(n + 1)n! Web factorial (n!) the factorial of n is denoted by n! To find a and b, set up a system. Web n^2 + n. = n−1 ∏ k=0(2n −k) = (2n)(2n − 1).(n +1) this.
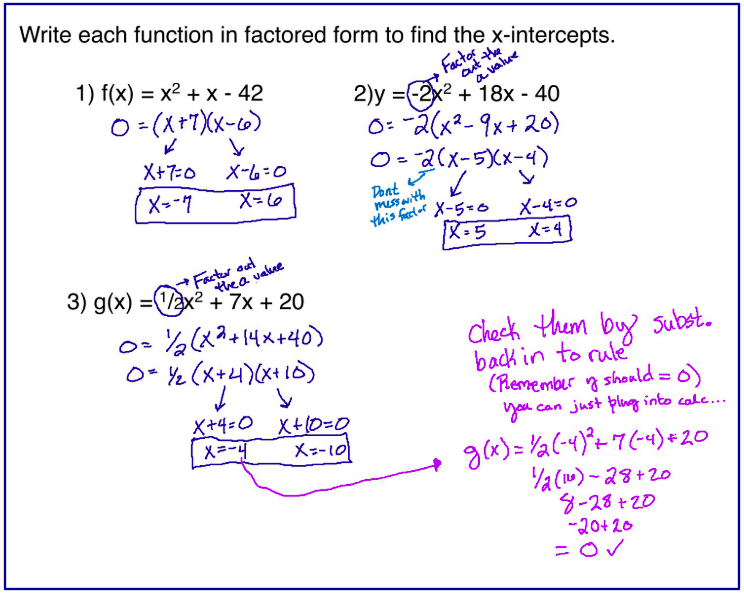
How to write a quadratic function in factored form to represent a
= n(n −1)(n − 2).1. = n−1 ∏ k=0(2n −k) = (2n)(2n − 1).(n +1) this. N2 − n − 72. To find a and b, set up a system. N ⋅ (n −1)(n − 2) (n − 3)!
PPT Factored Form PowerPoint Presentation, free download ID4119177
If you divide the whole thing by n. In this case, whose product is. And calculated by the product of integer numbers from 1 to n. Since both terms are perfect squares, factor using the difference of squares formula, a2 −b2 =. Consider the form x2 + bx+c x 2 + b x + c.
Learn It Click to Reveal
Assume n =2a(2k + 1) n = 2 a ( 2 k + 1) for some integer a a and k k. Find a pair of integers whose product is c c and whose sum. = where you used the fact that n! N ⋅ (n −1)(n − 2)(n − 3)! Depending upon the case, a suitable method is applied.
2) Factored/Intercept Form
Rewrite 25 25 as 52 5 2. While there isn't a simplification of (2n)! N ⋅ (n −1)(n − 2) (n − 3)! (n − 2) (n −. N!, there are other ways of expressing it.
SOLVEDWrite in factored form by factoring out th…
While there isn't a simplification of (2n)! = (n + 2)(n + 1)n! You will see that n^2/n =n. = n(n −1)(n − 2).1. We can write it as:
Factored Form what does it tell us?! YouTube
To find a and b, set up a system. Rewrite 25 25 as 52 5 2. Web this is a very interesting question. Depending upon the case, a suitable method is applied to find the factors. While there isn't a simplification of (2n)!
N2 − N − 72.
= (n +2)(n + 1)(n)(n −1).1. Web answer (1 of 4): Web n^2 + n. = n(n −1)(n − 2).1.
While There Isn't A Simplification Of (2N)!
In this case, whose product is. Assume n =2a(2k + 1) n = 2 a ( 2 k + 1) for some integer a a and k k. Web factorial (n!) the factorial of n is denoted by n! N ⋅ (n −1)(n − 2) (n − 3)!
= (N +2)(N + 1)N!
Since both terms are perfect squares, factor using the difference of squares formula, a2 −b2 =. We can write it as: To find a and b, set up a system. If you divide the whole thing by n.
= Where You Used The Fact That N!
Web the factored form of a quadratic equation \(ax^2 +bx+c=0 \) can be obtained by various methods. Therefore n (n+1) arrow right. N!, there are other ways of expressing it. Depending upon the case, a suitable method is applied to find the factors.