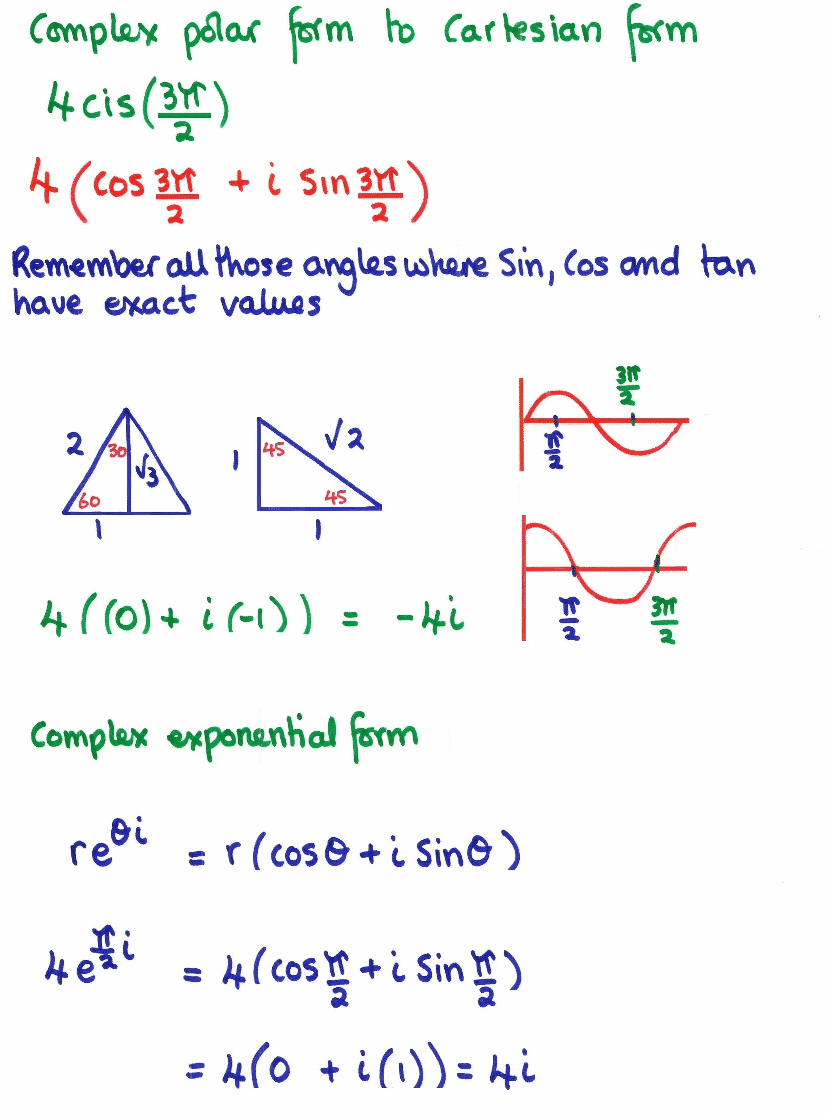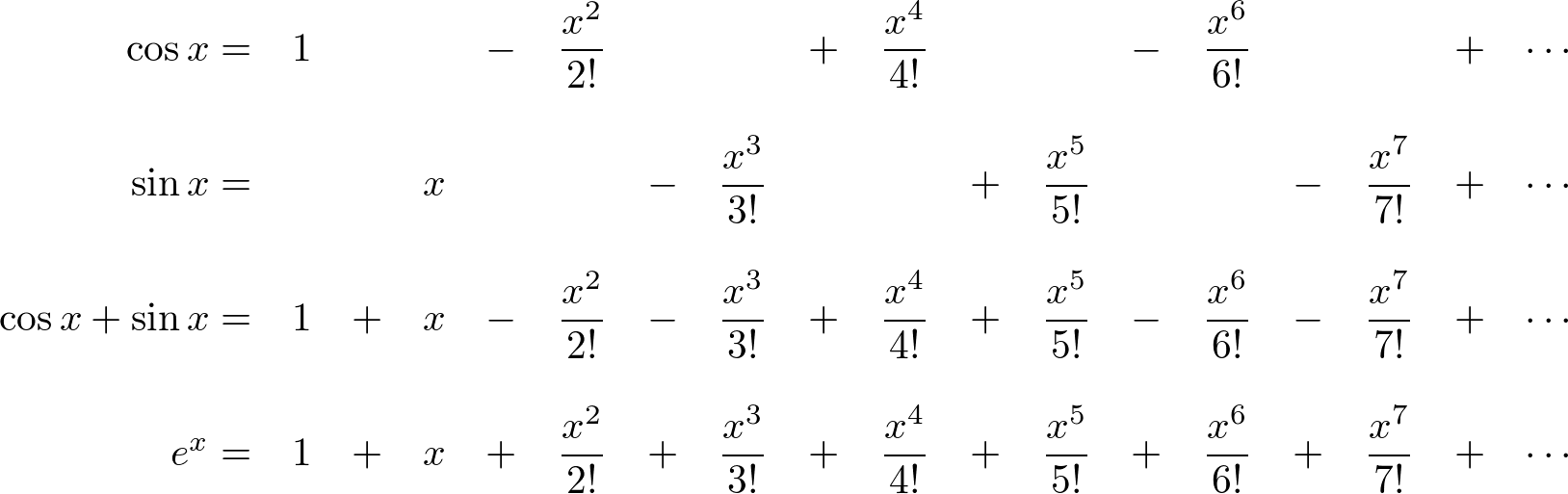Sin And Cos In Exponential Form
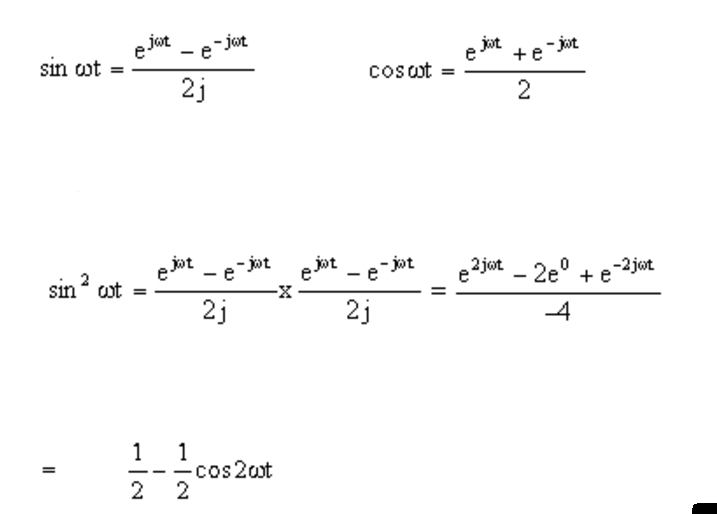
Sin And Cos In Exponential Form - Web notes on the complex exponential and sine functions (x1.5) i. Web we can use eulerβs theorem to express sine and cosine in terms of the complex exponential function as s i n c o s π = 1 2 π π β π , π = 1 2 π + π. Web we'll show here, without using any form of taylor's series, the expansion of \sin (\theta), \cos (\theta), \tan (\theta) sin(ΞΈ),cos(ΞΈ),tan(ΞΈ) in terms of \theta ΞΈ for small \theta ΞΈ. A) sin(x + y) = sin(x)cos(y) + cos(x)sin(y) and. Exercises with answers are at the bottom of the page. Web tutorial to find integrals involving the product of sin x or cos x with exponential functions. Using these formulas, we can. Eix = cos x + i sin x e i x = cos x + i sin x, and eβix = cos(βx) + i sin(βx) = cos x β i sin x e β i x = cos ( β x) + i sin ( β x) = cos x β i sin. Sinz = exp(iz) β exp( β iz) 2i. If ΞΌ r then eiΞΌ def = cos ΞΌ + i sin ΞΌ.
The reciprocal identities arise as ratios of sides in the triangles where this unit line. A) sin(x + y) = sin(x)cos(y) + cos(x)sin(y) and. Sinz = exp(iz) β exp( β iz) 2i. Eix = cos x + i sin x e i x = cos x + i sin x, and eβix = cos(βx) + i sin(βx) = cos x β i sin x e β i x = cos ( β x) + i sin ( β x) = cos x β i sin. Sinz denotes the complex sine function. All the integrals included in the. If ΞΌ r then eiΞΌ def = cos ΞΌ + i sin ΞΌ. E jx = cos (x) + jsin (x) and the exponential representations of sin & cos, which are derived from euler's formula: Web notes on the complex exponential and sine functions (x1.5) i. Web relations between cosine, sine and exponential functions.
Eit = cos t + i. A) sin(x + y) = sin(x)cos(y) + cos(x)sin(y) and. Eix = cos x + i sin x e i x = cos x + i sin x, and eβix = cos(βx) + i sin(βx) = cos x β i sin x e β i x = cos ( β x) + i sin ( β x) = cos x β i sin. Web relations between cosine, sine and exponential functions. Sinz denotes the complex sine function. All the integrals included in the. Web notes on the complex exponential and sine functions (x1.5) i. I denotes the inaginary unit. Expz denotes the exponential function. Using these formulas, we can.
Write Equations Of Sine Functions Using Properties Calculator
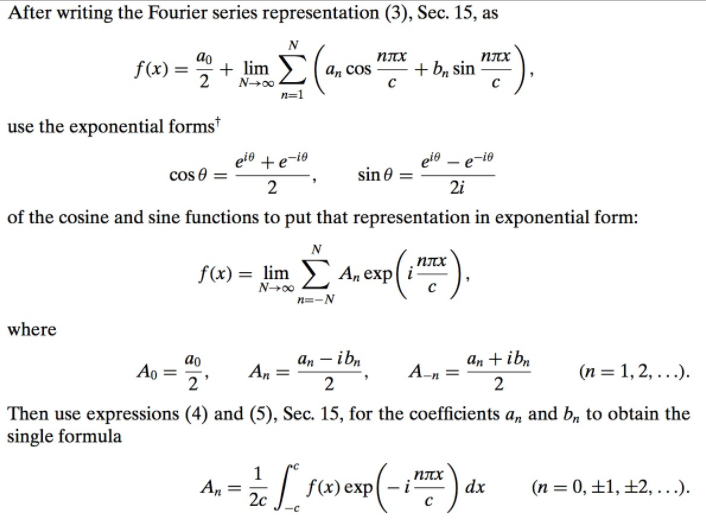
Web we can use eulerβs theorem to express sine and cosine in terms of the complex exponential function as s i n c o s π = 1 2 π π β π , π = 1 2 π + π. Intersection points of y=sin(x) and. Web using the exponential forms of cos(theta) and sin(theta) given in (3.11a, b), prove.
Basics of QPSK modulation and display of QPSK signals Electrical
A) sin(x + y) = sin(x)cos(y) + cos(x)sin(y) and. Using these formulas, we can. Rational expressions, equations, & functions. Eix = cos x + i sin x e i x = cos x + i sin x, and eβix = cos(βx) + i sin(βx) = cos x β i sin x e β i x = cos ( β x).
Other Math Archive January 29, 2018
Exercises with answers are at the bottom of the page. I denotes the inaginary unit. Web for any complex number z : Periodicity of the imaginary exponential. Expz denotes the exponential function.
Solving Exponential Trigonometric Equations 81^sin2x+81^cos^2x=30
The odd part of the exponential function, that is, sinh β‘ x = e x β e β x 2 = e 2 x β 1 2 e x = 1 β e β 2 x 2 e β x. Web relations between cosine, sine and exponential functions. E jx = cos (x) + jsin (x) and the exponential representations.
[Solved] I need help with this question Determine the Complex
Periodicity of the imaginary exponential. Sinz denotes the complex sine function. Web we can use eulerβs theorem to express sine and cosine in terms of the complex exponential function as s i n c o s π = 1 2 π π β π , π = 1 2 π + π. E jx = cos (x) + jsin (x).
Question Video Converting the Product of Complex Numbers in Polar Form
Web according to euler, we should regard the complex exponential eit as related to the trigonometric functions cos(t) and sin(t) via the following inspired definition: If ΞΌ r then eiΞΌ def = cos ΞΌ + i sin ΞΌ. Sinz = exp(iz) β exp( β iz) 2i. The odd part of the exponential function, that is, sinh β‘ x = e.
Relationship between sine, cosine and exponential function
Sinz = exp(iz) β exp( β iz) 2i. Web we'll show here, without using any form of taylor's series, the expansion of \sin (\theta), \cos (\theta), \tan (\theta) sin(ΞΈ),cos(ΞΈ),tan(ΞΈ) in terms of \theta ΞΈ for small \theta ΞΈ. Intersection points of y=sin(x) and. How to find out the sin value. The odd part of the exponential function, that is, sinh.
Question Video Evaluate a Definite Integral Involving the Exponential
How to find out the sin value. If ΞΌ r then eiΞΌ def = cos ΞΌ + i sin ΞΌ. Exercises with answers are at the bottom of the page. Web 1 answer sorted by: Eix = cos x + i sin x e i x = cos x + i sin x, and eβix = cos(βx) + i sin(βx).
Complex Polar and Exponential form to Cartesian
Expz denotes the exponential function. The odd part of the exponential function, that is, sinh β‘ x = e x β e β x 2 = e 2 x β 1 2 e x = 1 β e β 2 x 2 e β x. Web according to euler, we should regard the complex exponential eit as related to the.
Euler's Equation
Eit = cos t + i. How to find out the sin value. Web we can use eulerβs theorem to express sine and cosine in terms of the complex exponential function as s i n c o s π = 1 2 π π β π , π = 1 2 π + π. Web tutorial to find integrals involving.
Rational Expressions, Equations, & Functions.
All the integrals included in the. Web using the exponential forms of cos(theta) and sin(theta) given in (3.11a, b), prove the following trigonometric identities: Eit = cos t + i. Expz denotes the exponential function.
The Odd Part Of The Exponential Function, That Is, Sinh β‘ X = E X β E β X 2 = E 2 X β 1 2 E X = 1 β E β 2 X 2 E β X.
Sinz denotes the complex sine function. If ΞΌ r then eiΞΌ def = cos ΞΌ + i sin ΞΌ. Web 1 answer sorted by: Web exponential & logarithmic functions.
Web We'll Show Here, Without Using Any Form Of Taylor's Series, The Expansion Of \Sin (\Theta), \Cos (\Theta), \Tan (\Theta) Sin(Ξ),Cos(Ξ),Tan(Ξ) In Terms Of \Theta Ξ For Small \Theta Ξ.
Web notes on the complex exponential and sine functions (x1.5) i. Web according to euler, we should regard the complex exponential eit as related to the trigonometric functions cos(t) and sin(t) via the following inspired definition: Web we can use eulerβs theorem to express sine and cosine in terms of the complex exponential function as s i n c o s π = 1 2 π π β π , π = 1 2 π + π. Intersection points of y=sin(x) and.
The Reciprocal Identities Arise As Ratios Of Sides In The Triangles Where This Unit Line.
E jx = cos (x) + jsin (x) and the exponential representations of sin & cos, which are derived from euler's formula: Web tutorial to find integrals involving the product of sin x or cos x with exponential functions. Sinz = exp(iz) β exp( β iz) 2i. Eix = cos x + i sin x e i x = cos x + i sin x, and eβix = cos(βx) + i sin(βx) = cos x β i sin x e β i x = cos ( β x) + i sin ( β x) = cos x β i sin.