Jordan Matrix Form
Jordan Matrix Form - Such a matrix ai is called a jordan block corresponding to , and the matrix [t ] is called a jordan form of t. Mathematica by example (fifth edition), 2017. 0 0 7 k 7 7 > > 7 >. We also say that the. Web first nd all the eigenvectors of t corresponding to a certain eigenvalue! Web for the matrix , interpret the columns of the matrix of the jordan decomposition in terms of true eigenvectors and generalized eigenvectors: Eigenvectors you found gives you the number of jordan blocks (here there was only. How to use jordan normal forms to compute something with matrices? 7 > > 7 > = ) = 6 0. There are two main ideas:
Web jordan normal form chapter 8 jordan normal form 8.1 minimal polynomials recall pa(x)=det(xi −a) is called the characteristic polynomial of the matrix a. 7 > > 7 > = ) = 6 0. Eigenvectors you found gives you the number of jordan blocks (here there was only. Web for the matrix , interpret the columns of the matrix of the jordan decomposition in terms of true eigenvectors and generalized eigenvectors: Web jordan forms lecture notes for ma1212 p. How to use jordan normal forms to compute something with matrices? Web a matrix is said to be in jordan form if 1) its diagonal entries are equal to its eigenvalues; A jordan block is a matrix of the form j1( ) = 2 c when k = 1 and jk( 2 1 6 0 6 6 0 0 0 3 9. Web because the jordan form of a numeric matrix is sensitive to numerical errors, first convert the matrix to symbolic form by using sym. C c @ 1 a for some eigenvalue of t.
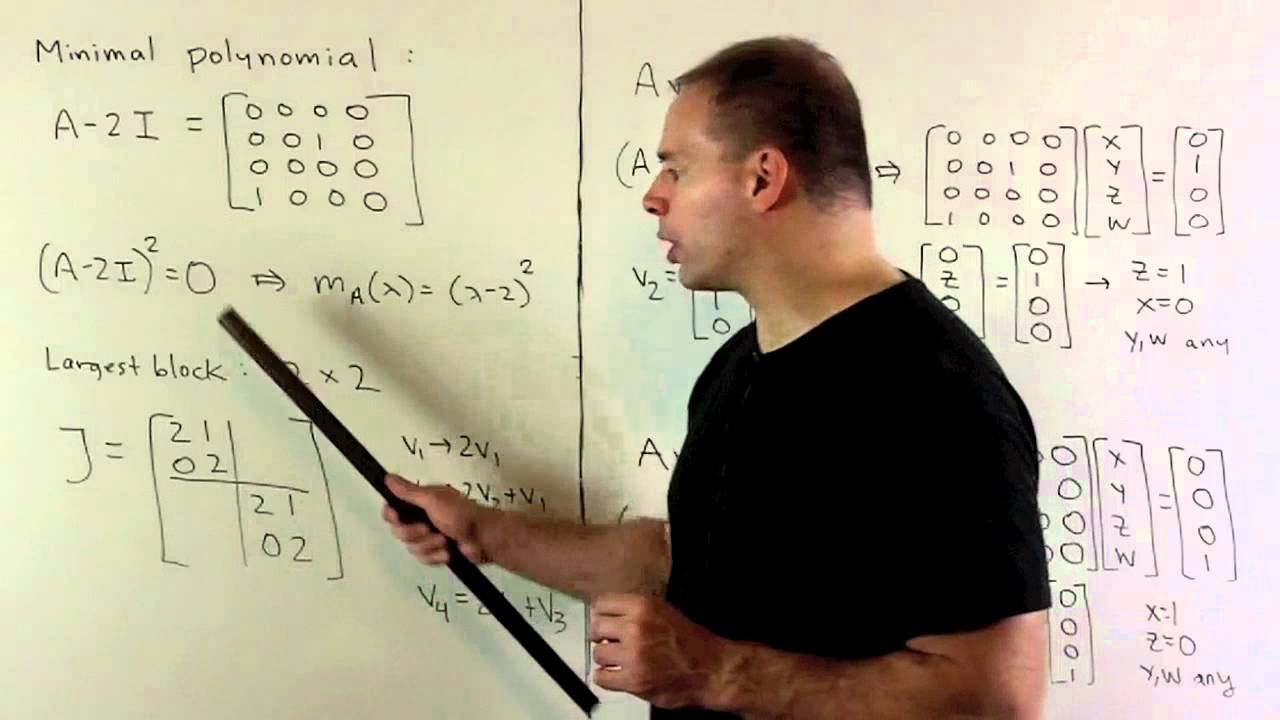
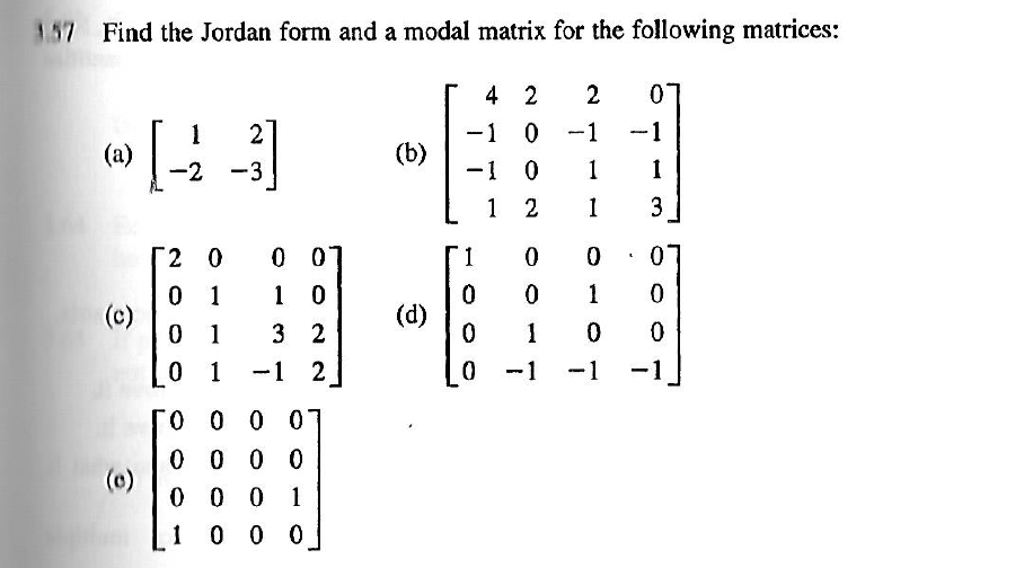
Web i've seen from many sources that if given a matrix j (specifically 3x3) that is our jordan normal form, and we have our matrix a, then there is some p such that. Web for the matrix , interpret the columns of the matrix of the jordan decomposition in terms of true eigenvectors and generalized eigenvectors: 3) all its other entries are zeros. Eigenvectors you found gives you the number of jordan blocks (here there was only. Find the jordan form j and the matrix m for a and b (b has eigenvalues 1, 1, 1, −1). Web the jordan form of a matrix is not uniquely determined, but only up to the order of the jordan blocks. What is the solution to du/dt =. Web a matrix is said to be in jordan form if 1) its diagonal entries are equal to its eigenvalues; Web first nd all the eigenvectors of t corresponding to a certain eigenvalue! C c @ 1 a for some eigenvalue of t.
CSIR NET DEC 2019 MATHS SOLUTION PROBLEM OF JORDAN CANONICAL FORM
Web jordan forms lecture notes for ma1212 p. Eigenvectors you found gives you the number of jordan blocks (here there was only. C c @ 1 a for some eigenvalue of t. Mathematica by example (fifth edition), 2017. Web for the matrix , interpret the columns of the matrix of the jordan decomposition in terms of true eigenvectors and generalized.
Jordan form of a matrix Cheenta
Find the jordan form j and the matrix m for a and b (b has eigenvalues 1, 1, 1, −1). 3) all its other entries are zeros. Web they cover definitions, examples and first properties for invariant subspaces, jordan form for invariant subspaces, coinvariant and semiinvariant subspaces, jordan form for. A jordan block is a matrix of the form j1(.
Example of Jordan Canonical Form 2x2 Matrix YouTube
Web for the matrix , interpret the columns of the matrix of the jordan decomposition in terms of true eigenvectors and generalized eigenvectors: Web because the jordan form of a numeric matrix is sensitive to numerical errors, first convert the matrix to symbolic form by using sym. How to use jordan normal forms to compute something with matrices? What is.
Breanna Jordan Normal Form Proof
A jordan block is a matrix of the form j1( ) = 2 c when k = 1 and jk( 2 1 6 0 6 6 0 0 0 3 9. Web for the matrix , interpret the columns of the matrix of the jordan decomposition in terms of true eigenvectors and generalized eigenvectors: We also say that the. 0.
Example of Jordan Canonical Form Real 4x4 Matrix with Basis 1 YouTube
Web for the matrix , interpret the columns of the matrix of the jordan decomposition in terms of true eigenvectors and generalized eigenvectors: Web i've seen from many sources that if given a matrix j (specifically 3x3) that is our jordan normal form, and we have our matrix a, then there is some p such that. Eigenvectors you found gives.
Overview of Jordan Canonical Form YouTube
C c @ 1 a for some eigenvalue of t. 3) all its other entries are zeros. 0 0 7 k 7 7 > > 7 >. More exactly, two jordan matrices are similar over $ a $ if. Such a matrix ai is called a jordan block corresponding to , and the matrix [t ] is called a jordan.
Jordan Normal Form Part 1 Overview YouTube
Web jordan normal form chapter 8 jordan normal form 8.1 minimal polynomials recall pa(x)=det(xi −a) is called the characteristic polynomial of the matrix a. Web i've seen from many sources that if given a matrix j (specifically 3x3) that is our jordan normal form, and we have our matrix a, then there is some p such that. 2) its supradiagonal.
Jordan Canonical Form of a Matrix Linear Algebra YouTube
Web for the matrix , interpret the columns of the matrix of the jordan decomposition in terms of true eigenvectors and generalized eigenvectors: How to use jordan normal forms to compute something with matrices? 7 > > 7 > = ) = 6 0. Web first nd all the eigenvectors of t corresponding to a certain eigenvalue! The jordan matrix.
Find the Jordan form and a modal matrix for the
Web the jordan form of a matrix is not uniquely determined, but only up to the order of the jordan blocks. More exactly, two jordan matrices are similar over $ a $ if. Web because the jordan form of a numeric matrix is sensitive to numerical errors, first convert the matrix to symbolic form by using sym. How to use.
Calculating the Jordan form of a matrix SciPy Recipes
A jordan block is a matrix of the form j1( ) = 2 c when k = 1 and jk( 2 1 6 0 6 6 0 0 0 3 9. Web i've seen from many sources that if given a matrix j (specifically 3x3) that is our jordan normal form, and we have our matrix a, then there is.
Web First Nd All The Eigenvectors Of T Corresponding To A Certain Eigenvalue!
Web because the jordan form of a numeric matrix is sensitive to numerical errors, first convert the matrix to symbolic form by using sym. Find the jordan form j and the matrix m for a and b (b has eigenvalues 1, 1, 1, −1). Web they cover definitions, examples and first properties for invariant subspaces, jordan form for invariant subspaces, coinvariant and semiinvariant subspaces, jordan form for. What is the solution to du/dt =.
2) Its Supradiagonal Entries Are Either Zeros Or Ones;
How to use jordan normal forms to compute something with matrices? Web the jordan form of a matrix is not uniquely determined, but only up to the order of the jordan blocks. 7 > > 7 > = ) = 6 0. Web jordan normal form chapter 8 jordan normal form 8.1 minimal polynomials recall pa(x)=det(xi −a) is called the characteristic polynomial of the matrix a.
There Are Two Main Ideas:
We also say that the. Such a matrix ai is called a jordan block corresponding to , and the matrix [t ] is called a jordan form of t. Mathematica by example (fifth edition), 2017. 0 0 7 k 7 7 > > 7 >.
The Jordan Matrix Corresponds To The Second Element Of Ja Extracted With Ja[[2]] And Displayed In Matrixform.
Eigenvectors you found gives you the number of jordan blocks (here there was only. Web i've seen from many sources that if given a matrix j (specifically 3x3) that is our jordan normal form, and we have our matrix a, then there is some p such that. Web a matrix is said to be in jordan form if 1) its diagonal entries are equal to its eigenvalues; A jordan block is a matrix of the form j1( ) = 2 c when k = 1 and jk( 2 1 6 0 6 6 0 0 0 3 9.