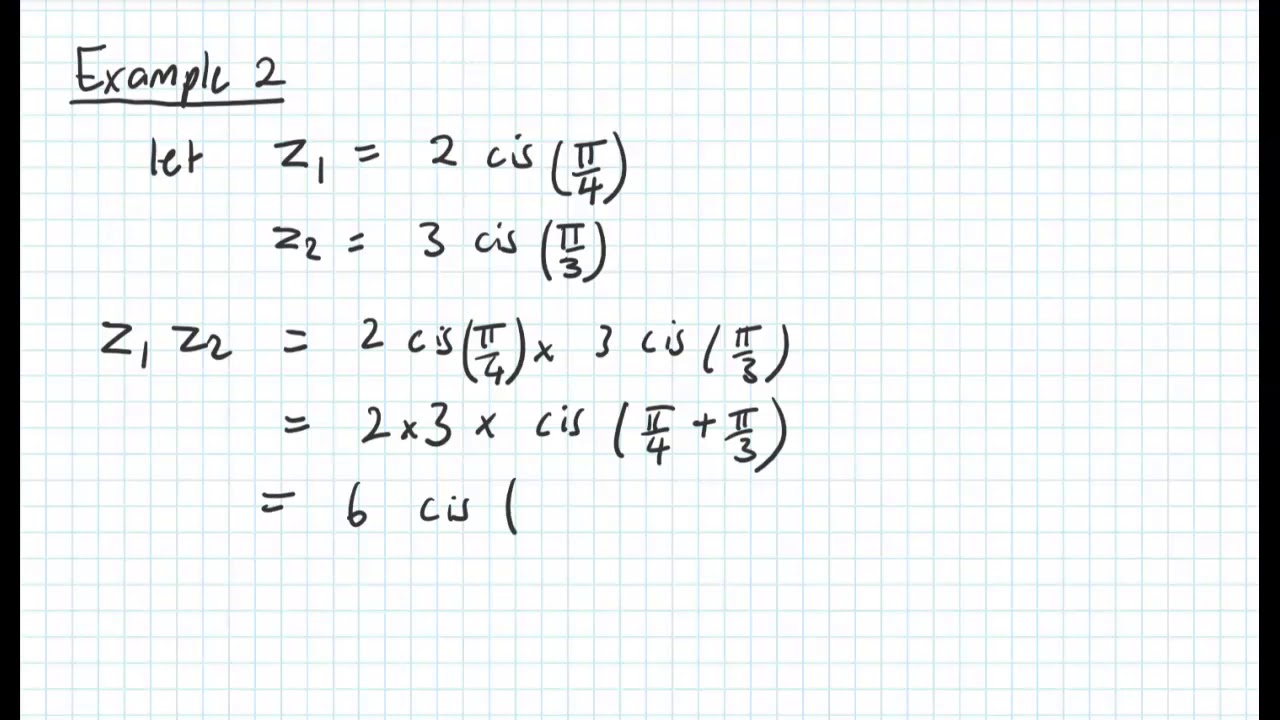How To Multiply Complex Numbers In Polar Form
How To Multiply Complex Numbers In Polar Form - Web in this video, i demonstrate how to multiply 2 complex numbers expressed in their polar forms. Multiplication of these two complex numbers can be found using the formula given below:. This video covers how to find the distance (r) and direction (theta) of the complex number on the complex plane, and how to use trigonometric functions and the pythagorean theorem to. Z1 ⋅ z2 = |z1 ⋅|z2| z 1 · z 2 = | z 1 · | z 2 |. And there you have the (ac − bd) + (ad + bc)i pattern. More specifically, for any two complex numbers, z 1 = r 1 ( c o s ( θ 1) + i s i n ( θ 1)) and z 2 = r 2 ( c o s ( θ 2) + i s i n ( θ 2)), we have: Sum the values of θ 1 and θ 2. Suppose z 1 = r 1 (cos θ 1 + i sin θ 1) and z 2 = r 2 (cos θ 2 + i sin θ 2) are two complex numbers in polar form, then the product, i.e. It is just the foil method after a little work: Web so by multiplying an imaginary number by j2 will rotate the vector by 180o anticlockwise, multiplying by j3 rotates it 270o and by j4 rotates it 360o or back to its original position.
This rule is certainly faster,. Web 2 answers sorted by: Sum the values of θ 1 and θ 2. To multiply complex numbers in polar form, multiply the magnitudes and add the angles. And there you have the (ac − bd) + (ad + bc)i pattern. Web in this video, i demonstrate how to multiply 2 complex numbers expressed in their polar forms. Web visualizing complex number multiplication. Multiply & divide complex numbers in polar form. Web i'll show here the algebraic demonstration of the multiplication and division in polar form, using the trigonometric identities, because not everyone looks at the tips and thanks tab. Z1 ⋅ z2 = |z1 ⋅|z2| z 1 · z 2 = | z 1 · | z 2 |.
The result is quite elegant and simpler than you think! (3 + 2 i) (1 + 7 i) = (3×1 − 2×7) + (3×7 + 2×1)i = −11 + 23i why does that rule work? Multiplication of these two complex numbers can be found using the formula given below:. Web the figure below shows the geometric multiplication of the complex numbers 2 +2i 2 + 2 i and 3+1i 3 + 1 i. This rule is certainly faster,. Z1 ⋅ z2 = |z1 ⋅|z2| z 1 · z 2 = | z 1 · | z 2 |. Web to add complex numbers in rectangular form, add the real components and add the imaginary components. Given two complex numbers in the polar form z 1 = r 1 ( cos ( θ 1) + i sin ( θ 1)) and z 2 = r 2 ( cos ( θ 2) +. Web learn how to convert a complex number from rectangular form to polar form. Web multiplication of complex numbers in polar form.
Multiplying Complex Numbers in Polar Form YouTube
Sum the values of θ 1 and θ 2. Substitute the products from step 1 and step 2 into the equation z p = z 1 z 2 = r 1 r 2 ( cos ( θ 1 + θ 2). More specifically, for any two complex numbers, z 1 = r 1 ( c o s ( θ 1).
Multiplying complex numbers (polar form) YouTube
Substitute the products from step 1 and step 2 into the equation z p = z 1 z 2 = r 1 r 2 ( cos ( θ 1 + θ 2). Web to add complex numbers in rectangular form, add the real components and add the imaginary components. Web multiplying complex numbers in polar form when you multiply two.
How to find the product Vtext multiply divide complex numbers polar
Multiplication by j10 or by j30 will cause the vector to rotate anticlockwise by the. Web the figure below shows the geometric multiplication of the complex numbers 2 +2i 2 + 2 i and 3+1i 3 + 1 i. Web to add complex numbers in rectangular form, add the real components and add the imaginary components. Web multiplication of complex.
Multiply Polar Form Complex Numbers YouTube
Web multiplying complex numbers in polar form when you multiply two complex numbers in polar form, z1=r1 (cos (θ1)+isin (θ1)) and z2=r2 (cos (θ2)+isin (θ2)), you can use the following formula to solve for their product: Web to add complex numbers in rectangular form, add the real components and add the imaginary components. Web 2 answers sorted by: Sum the.
Polar form Multiplication and division of complex numbers YouTube
Web learn how to convert a complex number from rectangular form to polar form. Z1z2=r1r2 (cos (θ1+θ2)+isin (θ1+θ2)) let's do. To multiply complex numbers in polar form, multiply the magnitudes and add the angles. Web 2 answers sorted by: Substitute the products from step 1 and step 2 into the equation z p = z 1 z 2 = r.
How to write a complex number in polar form YouTube
W1 = a*(cos(x) + i*sin(x)). To divide, divide the magnitudes and. But i also would like to know if it is really correct. Hernandez shows the proof of how to multiply complex number in polar form, and works. Multiply & divide complex numbers in polar form.
Complex Numbers Multiplying and Dividing in Polar Form, Ex 1 YouTube
Web i'll show here the algebraic demonstration of the multiplication and division in polar form, using the trigonometric identities, because not everyone looks at the tips and thanks tab. Web multiplication of complex numbers in polar form. (3 + 2 i) (1 + 7 i) = (3×1 − 2×7) + (3×7 + 2×1)i = −11 + 23i why does that.
Multiplying Complex Numbers in Polar Form YouTube
Substitute the products from step 1 and step 2 into the equation z p = z 1 z 2 = r 1 r 2 ( cos ( θ 1 + θ 2). But i also would like to know if it is really correct. Web so by multiplying an imaginary number by j2 will rotate the vector by 180o anticlockwise,.
Complex Numbers Multiplying in Polar Form YouTube
See example \(\pageindex{4}\) and example \(\pageindex{5}\). Hernandez shows the proof of how to multiply complex number in polar form, and works. 13 by multiplying things out as usual, you get [r1(cosθ1 + i sinθ1)][r2(cosθ2 + i sinθ2)] = r1r2(cosθ1 cosθ2 − sinθ1 sinθ2 + i[sinθ1 cosθ2 + sinθ2 cosθ1]). Web to write complex numbers in polar form, we use the.
How to Multiply Complex Numbers in Polar Form? YouTube
To divide, divide the magnitudes and. 1 2 3 4 1 2 3 4 5 6 7 8 9. Sum the values of θ 1 and θ 2. Z1z2=r1r2 (cos (θ1+θ2)+isin (θ1+θ2)) let's do. 13 by multiplying things out as usual, you get [r1(cosθ1 + i sinθ1)][r2(cosθ2 + i sinθ2)] = r1r2(cosθ1 cosθ2 − sinθ1 sinθ2 + i[sinθ1 cosθ2 +.
(3 + 2 I) (1 + 7 I) = (3×1 − 2×7) + (3×7 + 2×1)I = −11 + 23I Why Does That Rule Work?
Z1 ⋅ z2 = |z1 ⋅|z2| z 1 · z 2 = | z 1 · | z 2 |. But i also would like to know if it is really correct. It is just the foil method after a little work: Multiplication by j10 or by j30 will cause the vector to rotate anticlockwise by the.
This Rule Is Certainly Faster,.
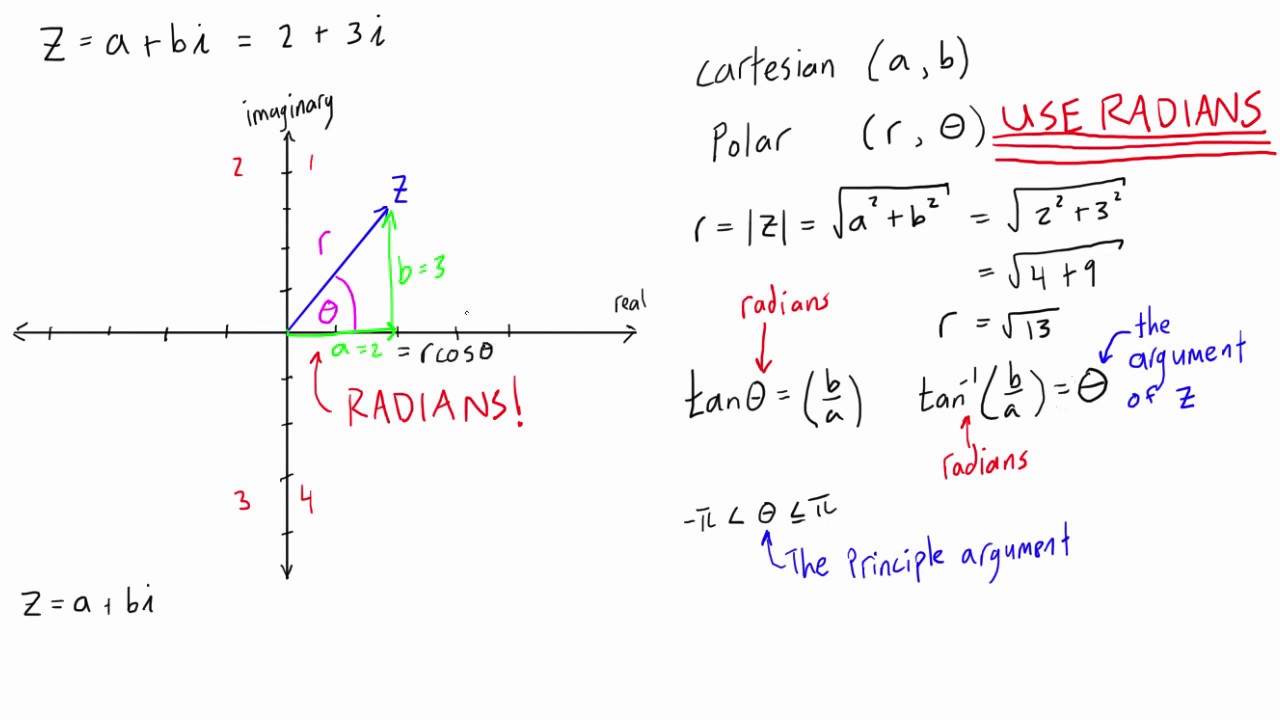
See example \(\pageindex{4}\) and example \(\pageindex{5}\). Web multiplication of complex numbers in polar form. Web to write complex numbers in polar form, we use the formulas \(x=r \cos \theta\), \(y=r \sin \theta\), and \(r=\sqrt{x^2+y^2}\). This video covers how to find the distance (r) and direction (theta) of the complex number on the complex plane, and how to use trigonometric functions and the pythagorean theorem to.
Multiplication Of These Two Complex Numbers Can Be Found Using The Formula Given Below:.
Substitute the products from step 1 and step 2 into the equation z p = z 1 z 2 = r 1 r 2 ( cos ( θ 1 + θ 2). Web multiplying complex numbers in polar form when you multiply two complex numbers in polar form, z1=r1 (cos (θ1)+isin (θ1)) and z2=r2 (cos (θ2)+isin (θ2)), you can use the following formula to solve for their product: Z1z2=r1r2 (cos (θ1+θ2)+isin (θ1+θ2)) let's do. Hernandez shows the proof of how to multiply complex number in polar form, and works.
Web 2 Answers Sorted By:
13 by multiplying things out as usual, you get [r1(cosθ1 + i sinθ1)][r2(cosθ2 + i sinθ2)] = r1r2(cosθ1 cosθ2 − sinθ1 sinθ2 + i[sinθ1 cosθ2 + sinθ2 cosθ1]). To convert from polar form to. For multiplication in polar form the following applies. More specifically, for any two complex numbers, z 1 = r 1 ( c o s ( θ 1) + i s i n ( θ 1)) and z 2 = r 2 ( c o s ( θ 2) + i s i n ( θ 2)), we have: