Fourier Series Exponential Form

Fourier Series Exponential Form - Web fourier series exponential form calculator. Web both the trigonometric and complex exponential fourier series provide us with representations of a class of functions of finite period in terms of sums over a. Web up to 5% cash back to represent the fourier series in concise form, the sine and cosine terms of trigonometric form, the fourier series are expressed in terms of exponential. Web complex exponential series for f(x) defined on [ − l, l]. Extended keyboard examples upload random. Web 1 answer sorted by: We can now use this complex exponential. 2 note that ∫π −πei(k−n)x dx = 2πδ −n ∫ − π π e i ( k − n) x d x = 2 π δ k − n i.e. Compute answers using wolfram's breakthrough. Web the complex exponential fourier seriesis a simple form, in which the orthogonal functions are the complex exponential functions.

The functions shown here are fairly simple, but the. } s(t) = ∞ ∑ k = −. Compute answers using wolfram's breakthrough. Web fourier series exponential form calculator. Web both the trigonometric and complex exponential fourier series provide us with representations of a class of functions of finite period in terms of sums over a. Web 1 answer sorted by: Web the complex exponential fourier series is the convenient and compact form of the fourier series, hence, its findsextensive application in communication theory. Web the trigonometric fourier series can be represented as: Using (3.17), (3.34a)can thus be transformed. F(t) = ao 2 + ∞ ∑ n = 1(ancos(nωot) + bnsin(nωot)) ⋯ (1) where an = 2 tto + t ∫ to f(t)cos(nωot)dt, n=0,1,2,⋯ (2) bn = 2 tto.

Web 1 answer sorted by: Web = 0 > 0 the response is an unbounded increasing exponential (blue line in plot) (image generated with this matlab script: Web the trigonometric fourier series can be represented as: Compute answers using wolfram's breakthrough. Web what we have studied so far are called real fourier series: 2 note that ∫π −πei(k−n)x dx = 2πδ −n ∫ − π π e i ( k − n) x d x = 2 π δ k − n i.e. F(x) ∼ ∞ ∑ n = − ∞cne − inπx / l, cn = 1 2l∫l − lf(x)einπx / ldx. Web complex exponential series for f(x) defined on [ − l, l]. Web for any periodic signal 𝑥 (𝑡), the exponential form of fourier series is given by, x ( t) = ∑ n = − ∞ ∞ c n e j n ω 0 t. We can now use this complex exponential.

PPT Lecture 11 PowerPoint Presentation, free download ID396272

Compute answers using wolfram's breakthrough. } s(t) = ∞ ∑ k = −. These decompose a given periodic function into terms of the form sin(nx) and cos(nx). Expon.m (matlab/expon.m) case when a is imaginary. K t, k = {., − 1, 0, 1,.

Solved 2. [45] Compute the exponential Fourier series

The functions shown here are fairly simple, but the. Compute answers using wolfram's breakthrough. F(x) ∼ ∞ ∑ n = − ∞cne − inπx / l, cn = 1 2l∫l − lf(x)einπx / ldx. Web the trigonometric fourier series can be represented as: Extended keyboard examples upload random.

Solved 2.18 Obtain the complex exponential Fourier series

We can now use this complex exponential. Web the trigonometric fourier series can be represented as: Web what we have studied so far are called real fourier series: Web = 0 > 0 the response is an unbounded increasing exponential (blue line in plot) (image generated with this matlab script: Web 1 answer sorted by:

Solved Exponential Fourier Series Example Find the complex

We can now use this complex exponential. Expon.m (matlab/expon.m) case when a is imaginary. Web what we have studied so far are called real fourier series: Web the complex exponential fourier series is the convenient and compact form of the fourier series, hence, its findsextensive application in communication theory. 2 note that ∫π −πei(k−n)x dx = 2πδ −n ∫ −.

Trigonometric Form Of Fourier Series

Compute answers using wolfram's breakthrough. ( 1) where, 𝜔 0 = 2𝜋⁄𝑇 is the angular frequency of the. Using (3.17), (3.34a)can thus be transformed. The functions shown here are fairly simple, but the. F(t) = ao 2 + ∞ ∑ n = 1(ancos(nωot) + bnsin(nωot)) ⋯ (1) where an = 2 tto + t ∫ to f(t)cos(nωot)dt, n=0,1,2,⋯ (2) bn.

PPT Chapter 7 Fourier Series PowerPoint Presentation, free download

We can now use this complex exponential. Web the complex exponential fourier seriesis a simple form, in which the orthogonal functions are the complex exponential functions. Web complex exponential series for f(x) defined on [ − l, l]. Web the complex fourier series expresses the signal as a superposition of complex exponentials having frequencies: Web what we have studied so.

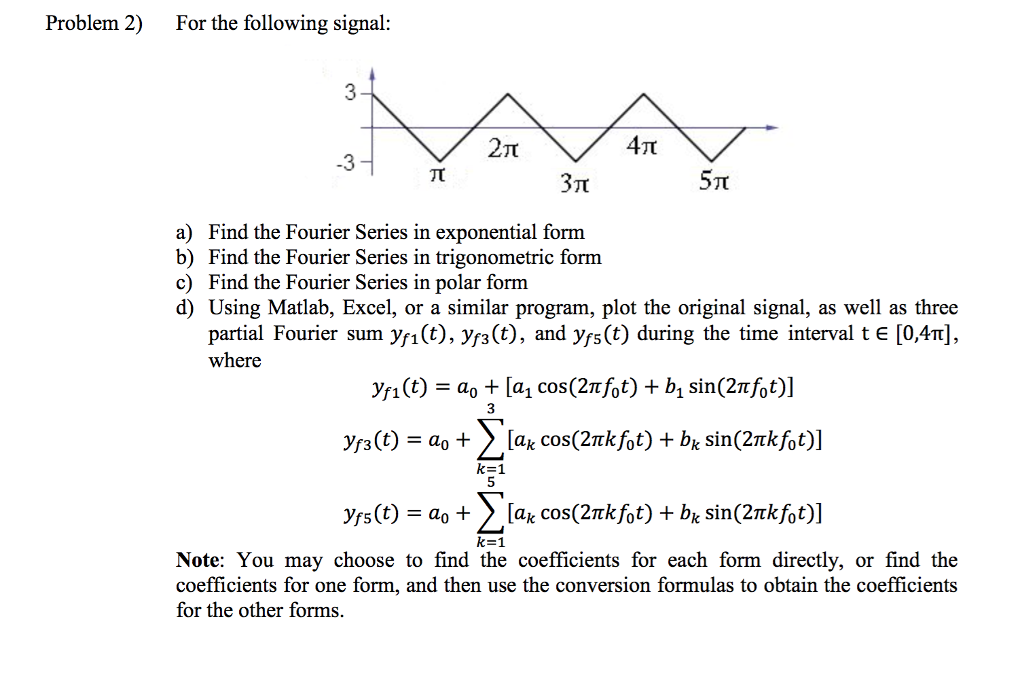
Solved Problem 2) For the following signal 4? 3 ? a) Find

These decompose a given periodic function into terms of the form sin(nx) and cos(nx). ( 1) where, 𝜔 0 = 2𝜋⁄𝑇 is the angular frequency of the. F(t) = ao 2 + ∞ ∑ n = 1(ancos(nωot) + bnsin(nωot)) ⋯ (1) where an = 2 tto + t ∫ to f(t)cos(nωot)dt, n=0,1,2,⋯ (2) bn = 2 tto. Web the trigonometric.

Complex Exponential Fourier Series YouTube

Compute answers using wolfram's breakthrough. Web both the trigonometric and complex exponential fourier series provide us with representations of a class of functions of finite period in terms of sums over a. F(x) ∼ ∞ ∑ n = − ∞cne − inπx / l, cn = 1 2l∫l − lf(x)einπx / ldx. Web what we have studied so far are.

Fourier Series Exponential Representation Mathematics Stack Exchange

Web 1 answer sorted by: Web the complex fourier series expresses the signal as a superposition of complex exponentials having frequencies: Web what we have studied so far are called real fourier series: Web the complex exponential fourier seriesis a simple form, in which the orthogonal functions are the complex exponential functions. Web complex exponential series for f(x) defined on.

Exponential Fourier Series Of Square Wave retpamind

F(x) ∼ ∞ ∑ n = − ∞cne − inπx / l, cn = 1 2l∫l − lf(x)einπx / ldx. Web complex exponential series for f(x) defined on [ − l, l]. Web fourier series directly from complex exponential form assume that f(t) is periodic in t and is composed of a weighted sum of harmonically related complex exponentials. Web.

Web 1 Answer Sorted By:

} s(t) = ∞ ∑ k = −. 2 note that ∫π −πei(k−n)x dx = 2πδ −n ∫ − π π e i ( k − n) x d x = 2 π δ k − n i.e. K t, k = {., − 1, 0, 1,. Web the trigonometric fourier series can be represented as:

Compute Answers Using Wolfram's Breakthrough.

Web what we have studied so far are called real fourier series: F(x) ∼ ∞ ∑ n = − ∞cne − inπx / l, cn = 1 2l∫l − lf(x)einπx / ldx. Extended keyboard examples upload random. Web fourier series exponential form calculator.

The Functions Shown Here Are Fairly Simple, But The.

Web both the trigonometric and complex exponential fourier series provide us with representations of a class of functions of finite period in terms of sums over a. Web for any periodic signal 𝑥 (𝑡), the exponential form of fourier series is given by, x ( t) = ∑ n = − ∞ ∞ c n e j n ω 0 t. ( 1) where, 𝜔 0 = 2𝜋⁄𝑇 is the angular frequency of the. Web the complex fourier series expresses the signal as a superposition of complex exponentials having frequencies:

Expon.m (Matlab/Expon.m) Case When A Is Imaginary.

Web = 0 > 0 the response is an unbounded increasing exponential (blue line in plot) (image generated with this matlab script: Web the complex exponential fourier seriesis a simple form, in which the orthogonal functions are the complex exponential functions. We can now use this complex exponential. These decompose a given periodic function into terms of the form sin(nx) and cos(nx).